Question

The angles in a triangle are such that one angle is 100 degrees more than the smallest angle, while the third angle is 2 times as large as the smallest angle. Find the measures of all three angles.

Answer 1

Explanation:

120,20 and 40

Answer 2

The measure of the three angles are 120°, 20° and 40°

Explanation:

Let

x ----> the measure of the first angle

y ---> the measure of the second angle (smallest angle)

z ---> the measure of the third angle

Remember that

The sum of the angles in a triangle must be equal to 180 degrees

so

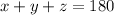`x+y+z=180` ----> equation A

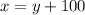`x=y+100` -----> equation B

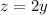`z=2y` ------> equation C

solve the system by substitution

substitute equation B and equation C in equation A

`(y+100)+y+(2y)=180`

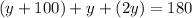
solve for y

`4y+100=180`

`4y=180-100`

`4y=80`

`y=20\°`

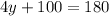
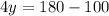
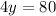
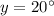
Find the value of x

`x=y+100` ----
`x=20+100=120\°`

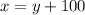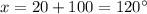
Find the value of z

`z=2y` ---->
`z=2(20)=40\°`

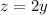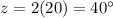
therefore

The measure of the three angles are 120°, 20° and 40°