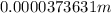Question

Interstellar space has an average temperature of about 10 K and an average density of hydrogen atoms of about one hydrogen atom per cubic meter. Calculate the mean free path of hydrogen atoms in interstellar space. Take d = 100 pm for a hydrogen atom.

Answer 1

The mean free path is
`0.0000373631 m`

Step-by-step explanation:

The formula for mean free path is :

λ =
`(V)/(√(2)\pi d^(2)N  )`

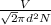
where,

λ - is the mean free path distance

V - volume of the gas

d - the diameter of the molecule

N - number of molecules.

now ,

density
`D` =
`(mass)/(volume)` =
`(M)/(V)` ;

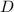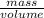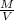mass of the gas = (number of molecules)
`*`(mass of one molecule) ;

as it's atomic hydrogen

`M = N*m \\m=1.66*10^(-24)\\M=N*1.66*10^(-24)`

∴

`D` =
`(N*1.66*10^(-24))/(V)`

∴

`(V)/(N*1.66*10^(-24)) = (1)/( D)`

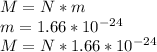
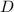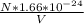
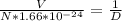
⇒ λ =
`(1)/(√(2)\pi d^(2)D  )`

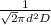
⇒ λ =
`(1.66*10^(-24))/(√(2)\pi (100*10^(-12))^(2)*1    )`

⇒ λ =
`0.0000373631 m`