Answer:
W=2.2 N
F=1.4 N
W'=0.8 N
Step-by-step explanation:
Given that
Radius ,r = 0.0135 m
Density of the platinum ,ρ₁ = 2.14 x 10⁴ kg/m³
Density of the mercury ,ρ₂ = 1.36 x 10⁴ kg/m³
The weight of the sphere
W= m g
mass = m = volume x density

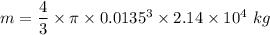
m = 0.22 kg
W= 0.22 x 10 = 2.2 N (↓) ( take g =10 m/s²)
The buoyant force
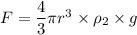
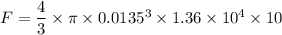
F= 1.4 N (↑)
The apparent weight
W' = 2.2 - 1.4 N
W'= 0.8 N