Question

1. A researcher is interested in knowing about the number of hours UCF students sleep per night. In a survey of 400 UCF students, the average number of hours slept per night was 6.5 with a population standard deviation of 2 hours. a. Create a 95% confidence interval for the true number of hours slept by UCF students?

Answer 1

Answer: (6.304, 6.696)

Explanation:

The confidence interval for population mean is given by :-

`\overline{x}\pm z^*(\sigma)/(√(n))`

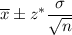
, where
`\sigma` = Population standard deviation.

n= sample size

`\overline{x}` = Sample mean

z* = Critical z-value .

Let x denotes the number of hours slept by UCF students.

Given :
`\sigma=2\ hours`

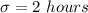
n= 400

`\overline{x}= 6.5\ hours`

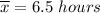
Two-tailed critical value for 95% confidence interval =
`z^*=1.96`

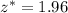
Then, the 95%confidence interval for the true number of hours slept by UCF students will be :-

`6.5\pm(1.96)(2)/(√(400))\\\\=6.5\pm(1.96)(2)/(20)\\\\=6.5\pm0.196=(6.5-0.196,\ 6.5+0.196)=(6.304,\ 6.696)`

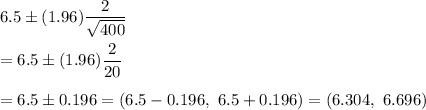
Hence, the 95% confidence interval for the true number of hours slept by UCF students : (6.304, 6.696)