Question

Consider the sequence:

5, 7, 11, 19, 35,....


Write an explicit definition that defines the sequence:


Group of answer choices


a_n=2n+3 a n = 2 n + 3


a_n=3n+2 a n = 3 n + 2


a_n=3n^2 a n = 3 n 2


a_n=2^n+3

Answer 1

`a_(n)=2^(n)+3`

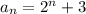
Explanation:

The given sequence is not arithmetic, it's a geometric sequence, that means the sequence is obtain using powers. The faster way to find the answer is to try options that fist with a geometric sequence. If we try the last one, we'll find that's the answer.

We need to try for n=1, n=2, n=3, n=4 and n=5:

a_{1}=2^{1}+3=5

a_{2}=2^{2}+3=4+3=7

a_{3}=2^{3}+3=8+3=11

a_{4}=2^{4}+3=16+3=19

a_{5}=2^{5}+3=32+3=35

Therefore, the right answer is the last choice, because as you can observe, it fits perfectly with the given sequence.