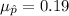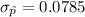Answer:
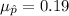
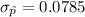
Explanation:
We know that the mean and the standard error of the sampling distribution of the sample proportions will be :-
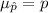
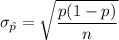
, where p=population proportion and n= sample size.
Given : The proportion of students at a college who have GPA higher than 3.5 is 19%.
i.e. p= 19%=0.19
The for sample size n= 25
The mean and the standard error of the sampling distribution of the sample proportions will be :-
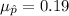

Hence , the mean and the standard error of the sampling distribution of the sample proportions :