Answer:
2 seconds
5.74165 seconds
-32 ft/s²
119.73 ft/s
Step-by-step explanation:
t = Time taken
u = Initial velocity
v = Final velocity
s = Displacement
a = Acceleration due to gravity = 32 ft/s²

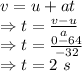
The maximum height will be reached in 2 seconds
From the given equation

The maximum height is 224 ft from the ground
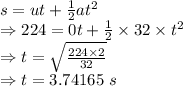
Time taken to fall from the maximum height is 3.74165 seconds
Time taken from the point in time the ball is thrown is 2+3.74165=5.74165 seconds
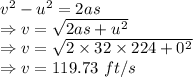
Velocity with which the ball will hit the ground is 119.73 ft/s
At t = 1
Acceleration of a body thrown is always i.e., a body in free fall is always 32 ft/s². Here as the ball is thrown up the it will be negative so -32 ft/s²