Question

11. Jillian needs to make a group of 5 people. She has 10 Democrats, 13 Republicans, and 7 Independents to choose from. (a) What is the probability that the group of 5 people will have 2 Democrats, 2 Republicans, and 1 Independent

Answer 1

0.1724 or 17.24%

Explanation:

There are

10+13+7 = 30 people

so there are C(30,5) combinations of 30 elements taken 5 at a time ways to select groups of 5 people.

`C(30,5)=\displaystyle\binom{30}{5}=(30!)/(5!25!)=142,506`

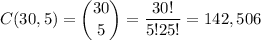
There are C(10,2) combinations of 10 elements taken 2 at a time ways to select the 2 Democrats

`C(10,2)=\displaystyle\binom{10}{2}=(10!)/(2!8!)=45`

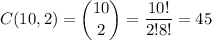
There are C(13,2) combinations of 13 elements taken 2 at a time ways to select the 2 Republicans

`C(13,2)=\displaystyle\binom{13}{2}=(13!)/(2!11!)=78`

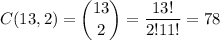
There are C(7,1) combinations of 7 elements taken 1 at a time ways to select the Independent

`C(7,1)=\displaystyle\binom{7}{1}=(7!)/(1!6!)=7`

By the Fundamental Principle of Counting, there are

45*78*7 = 24,570

ways of making the group of 5 people with 2 Democrats, 2 Republicans and 1 independent, and the probability of forming the group is

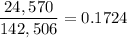`\displaystyle(24,570)/(142,506)=0.1724` or 17.24%