Question

a bullet is dropped from the same height when another bullet is fired horizontally they will hit the ground

Answer 1

At the same time.

Step-by-step explanation:

In the first case ,

intial velocity = 0

displacement = -h

acceleration = -g

Using second equation of motion,

s = ut + .5a
`t^(2) \\`

- h = - 0.5g
`t^(2) \\`

t =
`\sqrt{(2h)/(g) }`

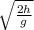
In the second case, consider only motion along y axis,

intial velocity = 0 ( all the velocity is along x axis)

displacement = -h ( height is same in both cases)

acceleration = -g

Using second equation of motion,

s = ut + .5a
`t^(2) \\`

- h = - 0.5g
`t^(2) \\`

t =
`\sqrt{(2h)/(g) }`

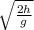
In both cases time is same.

Hence, they will reach the ground simultaneously.