The solution of (2.3 x 10^3) + (6.9 x 10^3) is
 that is 9200
that is 9200
Solution:
Need to solve the following expression:
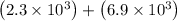
There are two terms in given expression
Let’s find GCF for this two terms
The greatest number that is a factor of two (or more) other numbers. When we find all the factors of two or more numbers, and some factors are the same ("common"), then the largest of those common factors is the Greatest Common Factor.
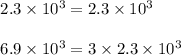
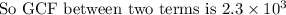
Let’s bring GCF out of the bracket in expression for sake of simplicity.
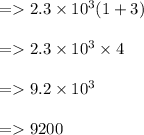
Hence on solving the expression (2.3 x 10^3) + (6.9 x 10^3) the result we get is
 that is 9200
that is 9200