Question

The equation 22 = 2y + x represents the perimeter of a flower garden with

length y (in feet) and width x (in feet). Solve for y. Then find the length of the
flower bed when the width is 2 feet, 3 feet, and 4 feet.

Answer 1

`y =  (22-x)/(2)`

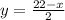
For width = 2 ft, the length of the flower bed = 10 ft.

For width = 3 ft, the length of the flower bed = 9.5 ft.

For width = 4 ft, the length of the flower bed = 9 ft.

Explanation:

Here, the Perimeter of the flower garden is given as

22 = 2 y + x

: where, y : Length of the garden

and x : Width of the garden .

Now, solving for y in the above expression,we get

22 = 2 y + x ⇒ 22 - x = 2 y

or,
`y =  (22-x)/(2)`

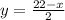
Now, when the width (x) = 2 feet

Length of the flower bed
`y =  (22-x)/(2)  = (22-2)/(2)  = (20)/(2)  = 10`

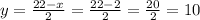
or, x = 10 ft

⇒For, the width = 2 ft, the length of the flower bed = 10 ft.

when the width (x) = 3 feet

Length of the flower bed
`y =  (22-x)/(2)  = (22-3)/(2)  = (19)/(2)  = 9.5`

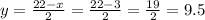
or, x = 9.5 ft

⇒For, the width = 3 ft, the length of the flower bed = 9.5 ft.

when the width (x) = 4 feet

Length of the flower bed
`y =  (22-x)/(2)  = (22-4)/(2)  = (18)/(2)  = 9`

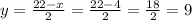
or, x = 9 ft

⇒For, the width = 4 ft, the length of the flower bed = 9 ft.