Question

A 12 cm x 2 cm rectangle sits inside a circle with radius of 8 cm.

What is the area of the shaded region?
Round your final answer to the nearest hundredth.

Answer 1

177.06

Explanation:

i got it wrong on khan academy

Answer 2

Diagram was not given.

Still I had drawn it, hope may it will helpful to you. So as per my diagram I had solved the sum.

The area of the shaded region is 176.96 ≈ 178 cm²

Explanation:

Given:

radius of the circle = r = 8 cm

length of the rectangle = L = 12 cm

breadth of the rectangle = B = 2 cm

To Find:

Area of shaded region = ?

Solution:

`\textrm{Area of rectangle} = Length* Breadth\\= L* B\\= 12* 2\\= 24\ cm^(2)`

`\textrm{Area of Circle} = \pi * (radius)^(2) \\=\pi * r^(2)\\=3.14* 8^(2)\\=200.96\ cm^(2)`

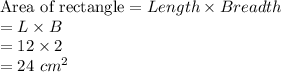
Now, The Area of Shaded region will be

`\textrm{Area of Shaded region}=\textrm{Area of Circle}-\textrm{Area of Rectangle}\\=200.96 - 24\\=176.96\ cm^(2) \\=178\ cm^(2)\........... \textrm{Rounded to tenth}`

The area of the shaded region is 176.96 ≈ 178 cm²