Answer:
The length of the rectangle garden is 20
 i.e 34.64 meters .
i.e 34.64 meters .
Explanation:
Given as :
The height of the pole = H = 30 m
The two angles of elevation as 60° and 30°
Let The length of the rectangle garden = L m
Now, From figure
The measure from pole ground to first elevation 60° = x m
The measure from pole ground to second elevation 30° = (L + x ) m
Now, from triangle BOC
Tan Ф =
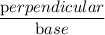
I.e Tan 60° =
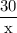
Or,
 =
=
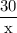
or, x =
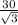
I.e x = 10
 meter ...1
meter ...1
Again From triangle BAC
Tan Ф =
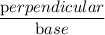
I.e Tan 30° =
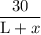
Or,
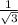 =
=
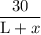
Or, L + x = 30 ×
 ....2
....2
Put the value of x from 1 into 2
i.e L + 10
 meter = 30 ×
meter = 30 ×

or, L = 30
 - 10
- 10

Or, L = 20
 = 34.64 meters
= 34.64 meters
I.e length of garden is 20

Hence The length of the rectangle garden is 20
 i.e 34.64 meters . answer
i.e 34.64 meters . answer