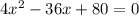The quadratic equation whose roots are 4 and 5, and whose leading coefficient is 4 is
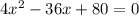
Solution:
Given that, roots of an quadratic equation are 4 and 5.
We have to find the equation of the quadratic equation.
Now, as 4 and 5 are roots, x = 4 and x = 5
Which means x – 4 and x – 5 are factors of the quadratic equation.
Then, equation will be the products of the factors
So, equation is (x - 4)(x - 5) = 0
Upon multiplication we get,
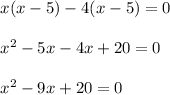
As we are given that, leading coefficient of the equation is 4. So multiply equation with 4
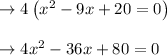
Hence, the quadratic equation is