Option 3
The solution for given expression is
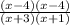
Solution:
Given that we have to divide,
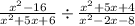 ---- (A)
---- (A)
Let us first factorize each term and then solve the sum
Using
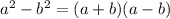
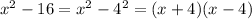 ----- (1)
----- (1)
 ----- (2)
----- (2)
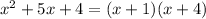 ---- (3)
---- (3)
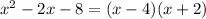 ---- (4)
---- (4)
Now substituting (1), (2), (3), (4) in (A) we get,
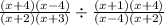
To do division with fractions, we turn the second fraction upside down and change the division symbol to a multiplication symbol at the same time. Then we treat this as a multiplication problem, by multiplying the numerators and the denominators separately.
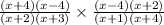
On cancelling terms we get,
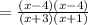
Thus option 3 is correct