Answer:
In the Pascal Expansion of
 the third term is
the third term is
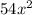 .
.
Explanation:
Here, the given expression is given as

Now, by the PASCAL'S TRIANGLE EXPANSION:
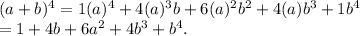
Substituting a = x and b = 3, we get:

In the given expansion, the third term is
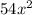 .
.
Hence, in the Pascal Expansion of
 the third term is
the third term is
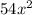 .
.