Question

in a laboratory the countof bacteria in a certain experiment was increasing at the rate of 5% per hour find the bacteria at the end of 3 hour if the count was initially 250000?​

Answer 1

289,406.25 ≈ 289,406

Step-by-step explanation:

We can solve this question in 2 ways: Simple calculation and Using the formula.

Simple Calculation: The rate of increase is 5% per hour. And the initial count was 250,000. Therefore, in the first hour, the increase in the count would be 5% of 250,00, which is
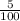`(5)/(100)`×250,000 = 12,500. Therefore, at the end of the first hour, the count would be 250,000 + 12500 = 262,500. Now, for the 2nd hour, the intial count is 262,500 and the increase in count at the end of the 2nd hour would be 5% of 262,500 =
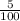`(5)/(100)`×262,500 = 13,125. And the count at the end of the 2nd hour is 262,500+13,125 = 275,625. Similarly for the 3rd hour, the starting count is 275,625 and the increase in count at the end of the 3rd hour is
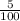`(5)/(100)`×275,625 = 13,781.25. Thus, the total count at the end of 3rd hour is 275,625+13,781.25 = 289,406.25 ≈ 289,406.

Direct Formula (Actually, it is the compound interest formula):
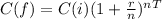`C(f) = C(i)(1 + (r)/(n))^(nT)` , where C(f) is final count, C(i) is the initial count, r is the rate of increase, n is the number of times rate of increase is applied in a time period, T is the number of time periods. Here, C(i) = 250,000, r is 5% = 0.05, n is one (because 5% increase happens once in one hour) and T is 3 hours. Therefore,
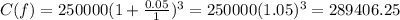`C(f) = 250000(1 + (0.05)/(1))^(3) = 250000(1.05)^(3) = 289406.25`.