Question

3x +10 < 3 or 2x -5 > 5

Answer 1

For this case we must find the solution set of the given inequalities:

Inequality 1:

`3x + 10 <3`

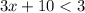
Subtracting 10 from both sides of the inequality:

`3x <3-10`

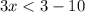
Different signs are subtracted and the major sign is placed.

`3x <-7`

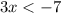
We divide between 3 on both sides of the inequality:

`x <- \frac {7} {3}`

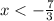
The solution is given by all values of x less than
`- \frac {7} {3}`

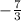
Inequality 2:

`2x-5> 5`

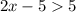
Adding 5 to both sides of the inequality:

`2x> 5 + 5\\2x> 10`

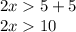
Dividing by 2 to both sides of the inequality:

`x> \frac {10} {2}\\x> 5`

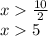
The solution is given by all values of x greater than 5.

Thus, the solution set is given by:

(-∞,
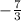`- \frac {7} {3}`) U (5,∞)

ANswer:

(-∞,
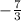`- \frac {7} {3}`) U (5,∞)