Answer:

Step-by-step explanation:
first write the newtons second law:
F
 =δma
=δma

Applying bernoulli,s equation as follows:
∑
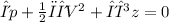
Where,
 is the pressure change across the streamline and
is the pressure change across the streamline and
 is the fluid particle velocity
is the fluid particle velocity
substitute
 for {tex]γ[/tex] and
for {tex]γ[/tex] and
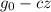 for
for

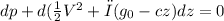
integrating the above equation using limits 1 and 2.
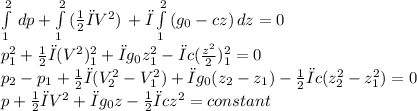
there the bernoulli equation for this flow is

note:
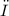 =density(ρ) in some parts and change(δ) in other parts of this equation. it just doesn't show up as that in formular
=density(ρ) in some parts and change(δ) in other parts of this equation. it just doesn't show up as that in formular