Question

A manufacturer of precision measuring instruments claims that the standarddeviation in the use of the instruments is less than 0.00002 millimeter. An analyst,who is unaware of claim, uses the instrument eight times and obtains a samplestandard deviation of 0.00001 millimeter. Assume normal population(i) Confirm using a test procedure and α level of 0.01 that there is insufficient evidence to support the claim that the standard deviation of the instruments is less than 0.00002 millimeters.(ii) Find the P-value for this test? How does it compare with α(greater than or less than)? Does it make sense with respect to your conclusionin part (i)?

Answer 1

i) There is insufficient evidence to support the claim that the standard deviation of the instruments is less than 0.00002 millimeters.

ii)
`p_v= P(\chi^2_(7) <1.75)=0.0276`

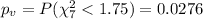
Explanation:

`s=0.00001` represent the sample standard deviation

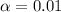`\alpha =0.01` represent the significance level for the test

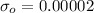`\sigma_o =0.00002` represent the value that we want to test

n=8 represent the sample size

A chi-square test can be used to test "if the standard deviation of a population is equal to a specified value. This test can be either a two-sided test or a one-sided test".

Null and alternatve hypothesis

The system of hypothesis on this case would be given by:

Null Hypothesis:
`\sigma \geq 0.00002`

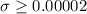
Alternative Hypothesis:
`\sigma <0.00002`

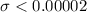
The statistic to test this is given by:

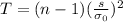`T=(n-1)((s)/(\sigma_0))^2` (1)

Part i) Calculate the statistic

If we replace into formula (1) we got this:

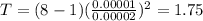`T=(8-1)((0.00001)/(0.00002))^2 =1.75` (1)

The critical region for a for a lower one-tailed alternative is given by

`T< \chi^2_(1-\alpha/2,N-1)`

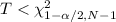
The degrees of freedom are given by

`df=n-1=8-1=7`

And if we use the Chi Square distribution with 7 degrees of freedom we see that
`\chi^2_(1-0.01/2,7)=1.239`

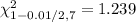
And our critical region would be
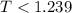`T< 1.239` so on this case we can conclude that we fail to reject the null hypothesis. So it's not enough evidence to conclude that the population standard deviation is less than 0.00002 mm.

Part ii) Calculate the p value

In order to calculate the p value we can do this:

`p_v= P(\chi^2_(7) <1.75)=0.0276`

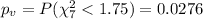
If we compare this value with the significance level (0.01) we see that
`p_v>\alpha`

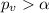
This agrees with the conclusion since when the p values is greater than the significance level we FAIL to reject the null hypothesis, same conclusion as part i).