Question

A man stands on a merry-go-round that is rotating at 1.58 rad/s. If the coefficient of static friction between the man's shoes and the merry-go-round is μs = 0.45, how far from the axis of rotation can he stand without sliding? (Enter the maximum distance in meters.) m

Answer 1

the maximum distance of rotation can he stand without sliding is 1.77 m

Step-by-step explanation:

given information:

angular velocity , ω = 1.58 rad/s

static friction, μs = 0.45

now we calculate the vertical force

N - W = 0, N is normal force and W is weight

N = W

= m g

next, for the horizontal force we only have frictional force, thus

F(friction) = m a

μs N = m a

μs m g = m a

a = μs g,

now we have to find the acceleration which is both translation and cantripetal.

a =
`\sqrt{a_(t) ^(2)+a_(c) ^(2)  }`

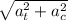
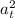`a_(t) ^(2)` is the acceleration for translation

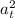`a_(t) ^(2)` = 0

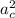`a_(c) ^(2)` is centripetal acceleration

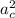`a_(c) ^(2)` = ω^2r

therefore,

a =
`\sqrt{a_(c) ^(2)  }`

=
`a_(c) ^(2)`

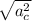
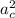
= ω^2r

Now, to find the radius, substitute the equation into the following formula

a = μs g

ω^2r = μs g

r = μs g / ω^2

= (0.45 x 9.8) / (1.58)

= 1.77 m