Answer:
Explanation:
Having drawn the line, Kendall must verify that the point P belongs to the line y = 2x-1 and then calculate the distance between A-P and verify if it is the closest to A or there is another one of the line
Having the point P(3,5) substitue x to verify y
y=2*(3)-1=6-1=5 (3,5)
Now if the angle formed by A and P is 90º it means that it is the closest point, otherwise that point must be found
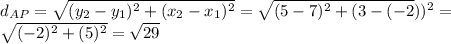
and we found the distance PQ and QA
;
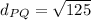 ,
,
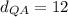
be the APQ triangle we must find <APQ through the cosine law (graph 2).