Question

The graph of function f is shown on the coordinate plane. Graph the line representing function g, if g is defined as shown below.

G(x)=1/2f(x+2)

Answer 1

`g(x)=x-1`

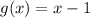
Explanation:

Any problem of this kind should first be approached by solving the initial function.

This question is based on curve translation on x-axis. But, there can be questions asked on translation in both axes, rotation of curve about origin, or both combined.

Initial function f(x) is a straight line from picture.

Common equation of straight line is

`y=mx+c`

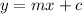
m= slope of the line

c= y-intercept

Slope of the line:

`m=(y_(2)-y_(1) )/(x_(2)-x_(1))`

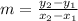
Let those points on graph be A(3,0) and B(0,-6)

Therefore, slope of line AB, using formula is:

`m=(0-(-6))/(3-0)`

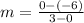
m=2

And c=(-6)

And equation of line AB is:

`y=2x-6`

`f(x)=2x-6`

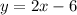
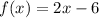
Now this line is translated backwards by 2 units, (x) becomes (x+2)

Let us consider a X, such that,
`X=x+2`

`f(X)=2X-6`

`f(x+2)=2(x+2)-6`

`f(x+2)=2x+4-6`

`f(x+2)=2x-2`

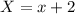
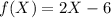
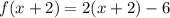
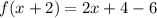
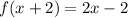
Now, there is another function such that,
`g(x)= (1)/(2) f(x+2)`

`g(x)= (1)/(2)(2x-2)`

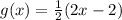
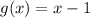`g(x)= x-1` ; according to your question.

In the picture, they are asking for a function,
`g(x)= (-1)/(2) f(x+2)`

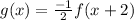
Then,
`g(x)= -x+1` ; according to the picture.

Here, I have attached a file showing all the graphs for clear understanding.

Answer 2

See attachment below.

Explanation:

f is a first-order polynomial, whose form is:

`y = m\cdot x + b`

Where:

`x` - Independent variable.

`y` - Dependent variable.

`m` - Slope.

`b` - y-Intercept.

The y-Intercept is equal to the value of the dependent variable when the independent variable is zero. Then:

`b = -6`

The slope is the ratio of the change in the dependent variable to the change in the independent variable. Hence:

`m =(\Delta y)/(\Delta x)`

`m = (0-(-3))/(3-0)`

`m = 1`

The equation for f is:

`f(x) = x -6`

The expression for g is found after substituting into and manipulating f:

`g(x) = -(1)/(2)\cdot [(x+2)-6]`

`g(x) = -(1)/(2)\cdot (x-4)`

`g(x) = -(1)/(2)\cdot x + 2`

The graph of the new function is plotted herein.