Question

A sample of 30 distance scores measured in yards has a mean of 7, a variance of 16, and a standard deviation of 4. You want to convert all your distances from yards to feet, so you multiply each score in the sample by 3. What are the new mean, median, variance, and standard deviation?

Answer 1

21, 144, 12

Explanation:

Given that a sample of 30 distance scores measured in yards has a mean of 7, a variance of 16, and a standard deviation of 4.

Let X be the distance in yard.

i.e. each entry of x is multiplied by 3.

New mean variance std devition would be

E(3x) =
`3E(x) = 21`

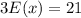
Var (3x) =
`3^2 Var(x) = 9(16) =144`

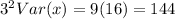
Std dev (3x) =
`√(144 ) =12`

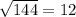
Thus we find mean and std devition get multiplied by 3, variance is multiplied by 9