To develop this problem it is necessary to apply the concepts related to conservation of the Moment and the cinematic equations of movement description. By definition we know that the conservation of the moment is given by

Where,
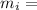 Mass
Mass
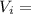 Velocity
Velocity
From the kinematic equations of motion we know that displacement as a function of acceleration (in this case gravity) is given by,
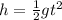
Where h is the height, g the gravity and t the time,
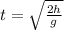
The horizontal velocity would be given as,
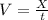
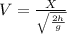
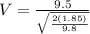
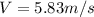
Replacing in our first equation we have that

Solving for

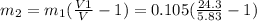
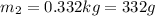
Therefore the apple had 332g.