Answer: The percent yield of the reaction is 61.5 %.
Step-by-step explanation:
To calculate the number of moles, we use the equation:
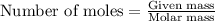 .....(1)
.....(1)
Given mass of butanol = 15.0 g
Molar mass of butanol = 74 g/mol
Putting values in equation 1, we get:

Given mass of NaBr = 22.4 g
Molar mass of NaBr = 103 g/mol
Putting values in equation 1, we get:
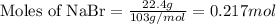
Given mass of sulfuric acid = 32.7 g
Molar mass of sulfuric acid = 98 g/mol
Putting values in equation 1, we get:

For the given chemical reaction:
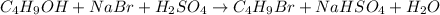
As, the reactants are present in 1 : 1 : 1 ratio. So, the reactant having minium number of moles will be considered as the limiting reagent.
Here, the limiting reagent is butanol because it limits the formation of product.
By Stoichiometry of the reaction:
1 mole of butanol produces 1 mole of bromobutane
So, 0.203 moles of butanol will produce =
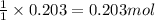 of bromobutane
of bromobutane
- Now, calculating the mass of bromobutane from equation 1, we get:
Molar mass of bromobutane = 137 g/mol
Moles of bromobutane = 0.203 moles
Putting values in equation 1, we get:

- To calculate the percentage yield of bromobutane, we use the equation:
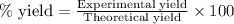
Experimental yield of bromobutane = 17.1 g
Theoretical yield of bromobutane = 27.81 g
Putting values in above equation, we get:
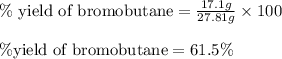
Hence, the percent yield of the reaction is 61.5 %.