Question

A student has a savings account earning 3% simple interest. She must pay $1900 for first-semester tuition by September 1 and $1900 for second-semester tuition by January 1. How much must she earn in the summer (by September 1) to pay the first-semester bill on time and still have the remainder of her summer earnings grow to $1900 between September 1 and January 1? (Round your answer to the nearest cent.)

Answer 1

$3781.19

Explanation:

Let us assume that the student has to earn $(1900 + x) by September 1 so that he can pay the $1900 tuition fee by September 1 and the remaining $x will grow at 3% simple interest to make him able to pay another tuition fee of $1900 by January 1.

So, we can write
`x( 1 + (4 * 3)/(12 * 100)) = 1900`

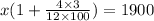
{Because September 1 to January 1 is 4 months and the monthly simple interest rate is
`(3)/(12)`%}

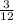⇒ 1.01x = 1900

⇒ x = $1881.19 (Rounded to the nearest cents)

Therefore, the student has to earn $(1900 + 1881.19) = $3781.19 (Answer)