Question

$12,400 is invested, part at 6% and the rest at 5%. If the interest earned from the amount invested at 6% exceeds the interest earned from the amount invested at 5% by $577.00, how much is invested at each rate? (Round to two decimal places if necessary.) Define variables x and y and set up a system of two linear equations that represents the information given in the problem.

Answer 1

Answer: $10881.81 is invested at 6% and $1518.18 is invested at 5%.

Explanation:

Since we have given that

Amount invested = $12400

Rate of interest for first part = 6%

Rate of interest for second part = 5%

Let the amount invested for 6% be 'x'.

Let the amount invested for 5% be 'y'

According to question, we get that

`0.06x-0.05y=577\\\\x+y=12400\\\\\implies x=12400-y`

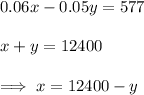
So, it becomes,

`0.06(12400-y)-0.05y=577\\\\744-0.06y-0.05y=577\\\\-0.11y=577-744\\\\-0.11y=-167\\\\y=(167)/(0.11)\\\\y=1518.18`

x=12400-y=12400-1518.18=$10881.81

Hence, $10881.81 is invested at 6% and $1518.18 is invested at 5%.