Question

10 kids are randomly grouped into an A team with five kids and a B team with five kids. Each grouping is equally likely.

(a) What is the size of the sample space?
(b) There are two kids in the group, Alex and his best friend Jose. What is the probability that Alex and Jose end up on the same team?

Answer 1

Answer: Our required probability is 0.44.

Explanation:

Since we have given that

Number of kids in team A = 5

Number of kids in team B = 5

(a) What is the size of the sample space?

So, the size of sample space would be

`(10!)/(5!* 5!)\\\\=252`

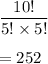
(b) There are two kids in the group, Alex and his best friend Jose. What is the probability that Alex and Jose end up on the same team?

Number of ways that Alex and Jose does not end up on same team would be

`(2!* 8!)/(4!* 4!)\\\\=140`

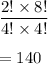
Number of ways that Alex and Jose end up on same team would be

252-140=112

So, the probability that Alex and Jose end up on the same team would be

`(112)/(252)=0.44`

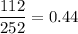
Hence, our required probability is 0.44.