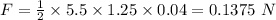Question

A metal disk of radius 4.0 cm is mounted on a frictionless axle. Current can flow through the axle out along the disk, to a sliding contact on the rim of the disk. A uniform magnetic field B= 1.25 T is parallel to the axle of the disk. When the current is 5.5 A, the disk rotates with constant angular velocity. What's the frictional force at the rim between the stationary electrical contact and t?

Answer 1

Friction force is 0.1375 N

Solution:

As per the question:

Radius of the metal disc, R = 4.0 cm = 0.04 m

Magnetic field, B = 1.25 T

Current, I = 5.5 A

Force on a current carrying conductor in a magnetic field is given by considering it on a differential element:

`dF = IB* dR`

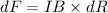
Integrating the above eqn:

`\int dF = IB\int_(0)^(R)dr`

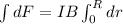
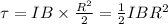`\tau = IB* (R^(2))/(2) = (1)/(2)IBR^(2)` (1)

Now the torque is given by:

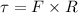`\tau = F* R` (2)

From eqn (1) and (2):

`F* R = (1)/(2)IBR^(2)`

Thus the Frictional force is given by:

`F = (1)/(2)* 5.5* 1.25* 0.04 = 0.1375\ N`