Question

After a completely inelastic collision, two objects of the same mass and same initial speed move away together at half their initial speed. Find the angle between the initial velocities of the objects.

Answer 1

120°

Step-by-step explanation:

given,

mass of two objects are same , Let it be m

speed of both the objects = v

after inelastic collision the

mass are moving with velocity = v/2

let the angle between them be θ₁ and θ₂

writing the equation along y-axis

m v sinθ₁ - m v sinθ₂ = 0

m v sinθ₁ = m v sinθ₂

θ₁ = θ₂ = θ

writing the equation along x- axis

m v cos θ + m v cos θ = 2 m V

v cos θ = v/2

`cos\theta = (1)/(2)`

`\theta = cos^(-1)((1)/(2))`

`\theta = 60^0`

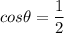
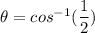
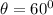
angle between the two objects = 2 θ

= 2 x 60° = 120°