Question

A computer manufacturer ships laptop computers with the batteries fully charged so that customers can begin to use their purchases right out of the box. In its last model, 85% of customers received fully charged batteries. To simulate arrivals, the company shipped 100 new model laptops (randomly picked from their warehouse) to various company sites around the country. Of 100 laptops shipped, 96 of them arrived reading 100% charged. Do the data provide evidence that this model’s rate is at least as high as the previous model? Test the hypothesis at α = 0.05.

Answer 1

The p value obtained and using the significance level assumed
`\alpha=0.05` we have
`p_v<\alpha` so we can conclude that we have enough evidenc to reject the null hypothesis, and we can said that at 5% of significance the proportion of new laptop's with fully charge for the new model is significantly higher compared to the old model.

Explanation:

1) Data given and notation n

n=100 represent the random sample taken

X=96 represent the laptop's arrived with fully charged batteries.

`\hat p=(96)/(100)=0.96` estimated proportion of laptop's arrived with fully charged batteries.

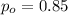`p_o=0.85` is the value that we want to test

`\alpha=0.05` represent the significance level

z would represent the statistic (variable of interest)

`p_v` represent the p value (variable of interest)

2) Concepts and formulas to use

We need to conduct a hypothesis in order to test the claim that the new model’s rate is at least as high as the previous model.:

Null hypothesis:
`p \leq 0.85`

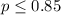
Alternative hypothesis:
`p > 0.85`

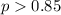
When we conduct a proportion test we need to use the z statisitc, and the is given by:

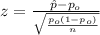`z=\frac{\hat p -p_o}{\sqrt{(p_o (1-p_o))/(n)}}` (1)

The One-Sample Proportion Test is used to assess whether a population proportion
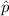`\hat p` is significantly different from a hypothesized value
`p_o`.

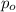3) Calculate the statistic

Since we have all the info requires we can replace in formula (1) like this:

`z=\frac{0.96 -0.85}{\sqrt{(0.85(1-0.85))/(100)}}=3.08`

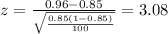
4) Statistical decision

P value method or p value approach . "This method consists on determining "likely" or "unlikely" by determining the probability assuming the null hypothesis were true of observing a more extreme test statistic in the direction of the alternative hypothesis than the one observed". Or in other words is just a method to have an statistical decision to fail to reject or reject the null hypothesis.

The significance level is not provided, but we can assume
`\alpha=0.05`. The next step would be calculate the p value for this test.

Since is a one side test the p value would be:

`p_v =P(z>3.08)=0.0010`

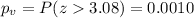
So based on the p value obtained and using the significance level assumed
`\alpha=0.05` we have
`p_v<\alpha` so we can conclude that we have enough evidenc to reject the null hypothesis, and we can said that at 5% of significance the proportion of new laptop's with fully charge for the new model is significantly higher compared to the old model.