Question

A triangular pyramid with a base area of 15 square inches is congruent to the base area of a hexagonal pyramid. Both pyramids have a height of 5. How do the volumes compare of the two solids? The hexagonal pyramid has a greater volume. The triangular pyramid has a greater volume. The hexagonal pyramid is 5 times the size of the triangular pyramid. The two pyramids have the same volume.

Answer 1

The two pyramids have the same volume.

Explanation:

What determines the volume of a pyramid is the Base Area, and its height. Since in these two figures, both bases measure the same (congruent) and their respective heights are equal. Then when we compare the volumes of these two solids, the triangular and the Hexagonal pyramid have the same volume, as it follows:

Then:

`\\V=(1)/(3)*B*h\\V_(\bigtriangleup \: Triangular\: base)=(1)/(3)*15*5\Rightarrow V_(\bigtriangleup \: pyramid)=15 \:in^(3)\\V_(\bigtriangleup \: hexagonal \:base)=(1)/(3)*15*5\Rightarrow V_(\bigtriangleup \: hexagonal \:base)=15 \:in^(3)`

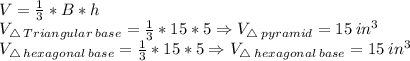
However, each surface area measure differently.