Question

Given aqueous solution of 0.1 M acetic acid of pka 4.72 and 0.1 M od sodium acettate, What volumes of actetic acid and sodium actetate should be mixed to make a 50 ml of a buffer soilution with ph 5.2?

Answer 1

12.44 mL of 0.1 M acetic acid solution

37.56 mL of 0.1 M sodium acetate solution

Step-by-step explanation:

To solve this problem we can use Henderson-Hasselbach's equation:

• pH = pKa + log [A⁻] / [HA]

Where [A⁻] and [HA] are the molar concentrations of sodium acetate and acetic acid, respectively.

First we use the equation to calculate the molar concentration ratio in the buffer solution:

• 5.2 = 4.72 + log [A⁻] / [HA]

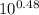• 0.48 = log [A⁻] / [HA]

• 
  `10^(0.48)` = [A⁻] / [HA]

• [A⁻] / [HA] = 3.02

We can rewrite the last part, using the definition of molar concentrations (C=n/V):

• 
  `(molAcetate/50 mL)/(molAcetic/50mL)` = 3.02

• 
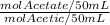  `(molAcetate)/(molAcetic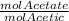)` = 3.02

From the problem we also know that:

• Vacetate + Vacetic = 50 mL

We rewirte the equation once again, keeping in mind that the moles of each substance come from the original 0.1 M solutions:

• molAcetate/Macetate + molAcetic/Macetic = 50 mL

• molAcetate/0.1 + molAcetic/0.1 = 0.050 L

So now we have a system of two equations and two unknowns, we use algebra to solve them:

• 
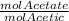  `(molAcetate)/(molAcetic)` = 3.02

• molAcetate = molAcetic * 3.02

We replace that value in the other equation:

• molAcetate/0.1 + molAcetic/0.1 = 0.050

• molAcetic * 3.02/0.1 + molAcetic/0.1 = 0.050

• 30.2 molAcetic + molAcetic/0.1 = 0.050

• 40.2 molAcetic = 0.050

• molAcetic = 1.243x10⁻³ mol

We use that value in the first equation:

• 
  `(molAcetate)/(molAcetic)` = 3.02

• 
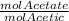  `(molAcetate)/(1.243*10^(-3) mol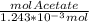)` = 3.02

• molAcetate = 3.754x10⁻³ mol

Finally, we use the definition of molarity to calculate the volume of each solution:

1. Acetic acid ⇒ 1.243x10⁻³ mol / 0.1 M = 0.0124 L = 12.44 mL
2. Acetate ⇒ 3.754x10⁻³ mol / 0.1 M = 0.0375 L = 37.56 mL