Answer:
57330.17766 Pa
Step-by-step explanation:
 = Pressure at 4000 m = 61660 Pa
= Pressure at 4000 m = 61660 Pa
 = Density at 4000 m = 0.8194 kg/m³
= Density at 4000 m = 0.8194 kg/m³
(Values taken from table of properties of air)
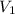 = Velocity of jet =
= Velocity of jet =
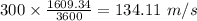
 = Velocity at max thickness
= Velocity at max thickness
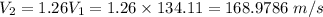
From Bernoulli's equation
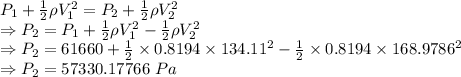
The absolute pressure at this point on the wing is 57330.17766 Pa