Answer:
0.9560
Explanation:
Given that based on historical data, your manager believes that 42% of the company's orders come from first-time customers
Population proportion p = 0.42
Sample size n = 85
p is Normal with mean = 0.42 and std error =
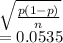
p is N(0.42, 0.0535)
Or we can say
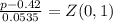
the probability that the sample proportion is between 0.36 and 0.47
=
