Answer:
C. y + 12 = -3(x – 5)
Explanation:
The point-slope form is
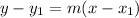
To express a line in the point-slope form, we actually its slope and a point that the line crosses. According to the problem, the point is
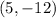 .
.
The slope we can find it from the expression that gives us the problem
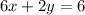
We are gonna express this equation in an explicit form, which is gonna give us the slope. Remember that in explicit form, the slope is the coefficient of the x-variable.

As you can observe, the slope is -3.
Now, using all this information, we can find the point-slope expression
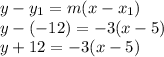
If we compare, we deduct that the correct answer is C., because is the same expression that we have.