Answer:
Explanation:
Hello!
Your study variable is X: scores obtained on mathematics SAT at the 2nd attempt of a high schooler.
This variable has a normal distribution and the population standard deviation is known σ= 47.53
Since you want to estimate the population mean, you have to work using the sample mean, its distribution is X[bar]~N(μ;σ²/n)
a.
Using the Z-table
[X[bar] ±
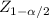 *(σ/√n)]
*(σ/√n)]
Where:
Z_{1-\alpha /2} = Z_{0.975} = 1.96
X[bar]= 20.99
n=380
[20.99 ±1.96*(47.53/√380)]
[16.2110;25.7689]
Using the 68-95-99.7 rule, since is based on the sample, the sample mean is x[bar] and the standard deviation for the sample mean distribution is: (σ/√n)
95% is between μ ± 2σ
Under the sample mean distribution:
mean: x[bar]= 20.99
standerd deviation: σ/√n = 47.53/√380
95% is between 20.99 ± 2*(47.53/√380) = 20.99 ± 2*2.4382
[16.1136;25.8664]
I hope it helps!