Answer:
B. (1, 0)
Explanation:
Given:
The two functions are:
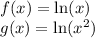
In order to determine the point of intersection of the graphs of the two given functions, we need to equate the functions.
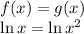
Two log functions with same base are equal only if their terms are equal to each other. Therefore,
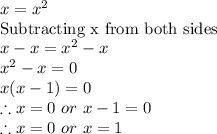
But a log function is not defined for
 . Therefore, the value of
. Therefore, the value of
 is only equal to 1.
is only equal to 1.
Now, the
 value can be obtained using any one of the function.
value can be obtained using any one of the function.
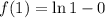 ( Since, log 1 = 0)
( Since, log 1 = 0)
Therefore, the point of intersection of the functions
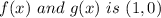 .
.
The correct option is B. (1, 0).