Question

A population of cats has a population mean of μ = 12μ=12pounds and a population standard deviation of σ = 2.0σ=2.0pounds. The distribution of weights of these cats is fairly symmetrical. If you take a random sample of 100 cats from this population, what will be the resulting sampling distribution of x?

Answer 1

Normal (12, 0.20)

Explanation:

Given that a population of cats has a population mean of

`\mu = 12pounds\\\sigma = 2 pounds`

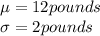
Also given that the distribution of weights of these cats is fairly symmetrical.

Since sample size is large and a random sample of 100 cats are drawn from this population, we find that

the mean of the sample would follow a normal distribution with mean =population mean and std deviation = population std dev/sqrt n

i.e. Sampling distribution of X would be normal with

mean = 12 pounds

and std deviation =
`(2)/(√(100) ) \\=0.20`