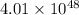Question

The universe is filled with photons left over from the Big Bang that today have an average energy of about 2 × 10−4 eV (corresponding to a temperature of 2.7 K). As derived in lecture, the number of available energy states per unit volume for photons is ????(????)????????

Answer 1

The number of available energy states per unit volume is
`4.01*10^(48)`

Step-by-step explanation:

Given that,

Average energy
`E=2*10^(-4)\ eV`

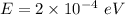
Photon =
`4*10^(-5)\ eV`

We need to calculate the number of available energy states per unit volume

Using formula of energy

`g(\epsilon)d\epsilon=(8\pi E^2dE)/((hc)^3)`

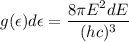
Where, E = energy

h = Planck constant

c = speed of light

Put the value into the formula

`g(\epsilon)d\epsilon=(8*\pi*2*10^(-4)*4*10^(-5)*1.6*10^(-19))/((6.67*10^(-34)*3*10^(8))^3)`

`g(\epsilon)d\epsilon=4.01*10^(48)`

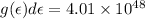
Hence, The number of available energy states per unit volume is
`4.01*10^(48)`