Question

(1 point) The effectiveness of a new bug repellent is tested on 18 subjects for a 10 hour period. Based on the number and location of the bug bites, the percentage of exposed surface area protected from bites was calculated for each of the subjects. Assume the population is normally distributed. The results were as follows: x¯¯¯=92 %, s=8% The new repellent is considered effective if it provides a percent repellency of more than 89%. Using α=0.025, construct a hypothesis test with null hypothesis μ≤0.89 and alternative hypothesis μ>0.89 to determine whether the mean repellency of the new bug repellent is greater than 89% by computing the following: (a)The degree of freedom is df= .

Answer 1

z=1.59

If we compare the p value and the significance level given
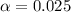`\alpha=0.025` we see that
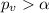`p_v>\alpha` so we can conclude that we fail to reject the null hypothesis, so we can conclude that the mean repellency of the new bug repellent is greater than 89% at 0.025 of signficance.

Step-by-step explanation:

1) Data given and notation

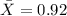`\bar X=0.92` represent the mean effectiveness of a new bug repellent for the sample

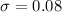`\sigma=0.08` represent the population standard deviation for the sample

`n=18` sample size

`\mu_o =0.89` represent the value that we want to test

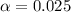`\alpha=0.025` represent the significance level for the hypothesis test.

t would represent the statistic (variable of interest)

`p_v` represent the p value for the test (variable of interest)

State the null and alternative hypotheses.

We need to conduct a hypothesis in order to determine if the mean repellency of the new bug repellent is greater than 89% or 0.89, the system of hypothesis would be:

Null hypothesis:
`\mu \leq 0.89`

Alternative hypothesis:
`\mu > 0.89`

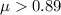
We don't know the population deviation, and the sample size <30, so for this case is better apply a t test to compare the actual mean to the reference value, and the statistic is given by:

`t=(\bar X-\mu_o)/((s)/(√(n)))` (1)

t-test: "Is used to compare group means. Is one of the most common tests and is used to determine if the mean is (higher, less or not equal) to an specified value".

Calculate the statistic

We can replace in formula (1) the info given like this:

`t=(0.92-0.89)/((0.08)/(√(18)))=1.59`

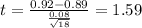
Calculate the P-value

First we need to calculate the degrees of freedom given by:

`df=n-1=18-1=17`

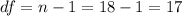
Since is a one-side upper test the p value would be:

`p_v =P(t_(17)>1.59)=0.065`

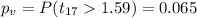
In Excel we can use the following formula to find the p value "=1-T.DIST(1.59;17;TRUE)"

Conclusion

If we compare the p value and the significance level given
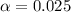`\alpha=0.025` we see that
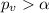`p_v>\alpha` so we can conclude that we fail to reject the null hypothesis, so we can conclude that the mean repellency of the new bug repellent is greater than 89% at 0.025 of signficance.