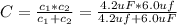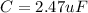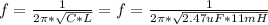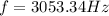Question

To construct an oscillating LC system, you can choose from a 11 mH inductor, a 6.0 μF capacitor, and a 4.2 μF capacitor. What are the (a) smallest, (b) second smallest, (c) second largest, and (d) largest oscillation frequency that can be set up by these elements in various combinations

Answer 1

a. 475.14 Hz

b. 1959 Hz

c. 2341.53 Hz , 3053.34 Hz

Step-by-step explanation:

`f = (1)/(2\pi*√(C*L))`

a. smallest use the capacitive 4.2 uF + 6.0 uF = 10.2uF replacing:

`f = (1)/(2\pi*√(C*L))f=(1)/(2\pi*√(10.2uF*11mH))`

`f = 475.14 Hz`

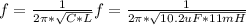
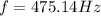
b. second smallest use the capacitive 6 uF so:

`f = (1)/(2\pi*√(C*L))=f = (1)/(2\pi*√(6uF*11mH))`

`f = 1959Hz`

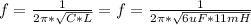
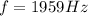
c. second largest and largest oscillation first combination so:

Use 4.2 uF

`f = (1)/(2\pi*√(C*L))=f = (1)/(2\pi*√(4.2uF*11mH))`

`f = 2341.53 Hz`

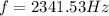
And finally largest oscillation cap in serie so:

`C=(c_1*c_2)/(c_1+c_2)=(4.2uF*6.0uf)/(4.2uf+6.0uF)`

`C=2.47 uF`

`f = (1)/(2\pi*√(C*L))=f = (1)/(2\pi*√(2.47uF*11mH))`

`f =  3053.34 Hz`