Question

Find the y intersept of

y=logbase (b)(a-k) based on the constants b and k ?

Answer 1

`(0, log_(b)(-k)), k < 0`

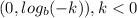
Explanation:

`y = \log_(b)(a - k)`

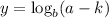
To find the y-intercept, set a = 0 and solve for y.

`y = \log_(b)(0 - k)\\y = \log_(b)(-k)`

This equation is undefined for k ≥ 0.

There is a y-intercept only if k < 0. Only then can the argument of the log function be positive.

The y-intercept is at

`\mathbf{(0, log_(b)(-k)), k < 0}`

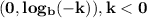
For example, if b = 2 and k = -3, log₂(3) = 1.585

The intercept is at (0, 1.585).