Question

A spherical balloon is made from a material whose mass is 3.30 kg. The thickness of the material is negligible compared to the 1.25 m radius of the balloon. The balloon is filled with helium (He) at a temperature of 345 K and just floats in air, neither rising nor falling. The density of the surrounding air is 1.19 kg/m³ and the molar mass of helium is 4.0026×10-3 kg/mol. Find the absolute pressure of the helium gas.

Answer 1

563712.04903 Pa

Step-by-step explanation:

m = Mass of material = 3.3 kg

r = Radius of sphere = 1.25 m

v = Volume of balloon =
`(4)/(3)\pi r^3`

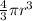
M = Molar mass of helium =
`4.0026* 10^(-3)\ kg/mol`

`\rho` = Density of surrounding air =
`1.19\ kg/m^3`

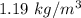
R = Gas constant = 8.314 J/mol K

T = Temperature = 345 K

Weight of balloon + Weight of helium = Weight of air displaced

`mg+m_(He)g=\rho vg\\\Rightarrow m_(He)=\rho vg-m\\\Rightarrow m_(He)=1.19* (4)/(3)\pi 1.25^3-3.3\\\Rightarrow m_(He)=6.4356\ kg`

Mass of helium is 6.4356 kg

Moles of helium

`n=(m)/(M)\\\Rightarrow n=(6.4356)/(4.0026* 10^(-3))\\\Rightarrow n=1607.85489`

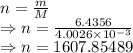
Ideal gas law

`P=(nRT)/(v)\\\Rightarrow P=(1607.85489* 8.314* 345)/((4)/(3)\pi 1.25^3)\\\Rightarrow P=563712.04903\ Pa`

The absolute pressure of the Helium gas is 563712.04903 Pa