Question

Seven points are marked on the circumference of a circle. How many different chords can be drawn by connecting two of these seven points?

Answer 1

21

Explanation:

In order to form a chord you need to:

1. Select one point. You have 7 choices
2. Select a another point. You have just 6 choices because you have to dicard the point alredy chosen
3. Connect the two points with a chorde

Since we have 7 choices for the first point and 6 for the second one, one might think that there are a total of 7*6 = 42 choices.

However the correct answer is 21, because you have to take into the account that selecting the point A first and selecting the point B on second step is the same than selecting the point B first and then the point A, because the chord is the same.

Therefore we are counting each chord twice, and we have to divide the result by 2, obtaining 7*6 / 2 = 21.

You can also calculate the amount by using the combiatorial number. The total amount of possibilities to select k elements from a set of n, with n higher than or equal to k and both positive, without taking into account the order is the combinatorial number of n with k, given by

`{n \choose k} = (n!)/(k!(n-k)!)`

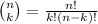
In this case, in order to form a chord we need to select 2 points of the seven we are given, ignoring order, thus the total amount of possibilities is

`{7 \choose 2} = (7!)/(2!5!) = (7*6*5*4*3*2*1)/(2*1*5*4*3*2*1) = (7*6)/(2) = 21`

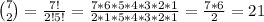
As we calculated before.