Question

If a radioactive isotope has a half-life of 26.5 days, how many days does it take for a sample of the isotope to decrease by 35.0% of its original value? View Available Hint(s) If a radioactive isotope has a half-life of 26.5 days, how many days does it take for a sample of the isotope to decrease by 35.0% of its original value? 40.1 days 16.5 days 7.15 days 0.0163 days

Answer 1

16.5 days

Step-by-step explanation:

Given that:

Half life = 26.5 days

`t_(1/2)=\frac {ln\ 2}{k}`

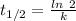
Where, k is rate constant

So,

`k=\frac {ln\ 2}{t_(1/2)}`

`k=\frac {ln\ 2}{26.5}\ days^(-1)`

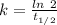
The rate constant, k = 0.02616 days⁻¹

Using integrated rate law for first order kinetics as:

`[A_t]=[A_0]e^(-kt)`

Where,

`[A_t]` is the concentration at time t

`[A_0]` is the initial concentration

Given:

35.0 % is decomposed which means that 0.35 of
`[A_0]` is decomposed. So,

`\frac {[A_t]}{[A_0]}` = 1 - 0.35 = 0.65

t = 7.8 min

`\frac {[A_t]}{[A_0]}=e^(-k* t)`

`0.65=e^(-0.02616* t)`

t = 16.5 days.