Question

Let the masses of blocks A and B be 4.50 kg and 2.00 kg , respectively, the moment of inertia of the wheel about its axis be 0.400 kg⋅m2, and the radius of the wheel be 0.100 m . Find the magnitude of linear acceleration of block A if there is no slipping between the cord and the surface of the wheel.A. A of Block A

B. A of Block B
C. Alpha of pulley
D. Tension side A
E. Tension Side B

Answer 1

Accelerations of both the sides is 0.6125
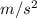`m/s^(2)`, A moves downwards whereas B moves upwards.

`\alpha`=6.125 rad/
`s^(2)`

Tension on side A = 4.5 × g= 44.1 m/
`s^(2)`

Tension on side B= 2.0 × g= 19.6 m/
`s^(2)`

Step-by-step explanation:

As both, the blocks A and B are attached due to the constraint they can only possess a single acceleration a.

Observe the figure attached, let the tension with Block A be
`T_(2)` and the tension attached with Block B be
`T_(1)` .

Tensions will be only be due to the weight of the blocks as no other force is present.

`T_(2)` = 4.5 × g= 44.1 m/
`s^(2)`

`T_(1)` = 2.0 × g= 19.6 m/
`s^(2)`

Now, lets make a torque equation about the center of the wheel and find the alpha

`T_(2)`×R-
`T_(1)`×R= MI( Moment of Inertia of Wheel)× Alpha

where, R= Radius of the wheel=0.100m and

Alpha(
`\alpha`)= Angular acceleration of the wheel

MI of the wheel= 0.400 kg/
`m^(2)`

`(44.1-19.6)R=0.400\alpha`

`\alpha = (24.5 * 0.100)/(0.400)`

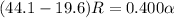
`\alpha`=6.125 rad/
`s^(2)`

Acceleration = R ×
`\alpha`

= 0.1 * 6.125

=0.6125
`m/s^(2)`

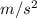
Accelerations of both the sides is 0.6125
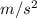`m/s^(2)`, A moves downwards whereas B moves upwards.