Question

The perimeter of a rectangle is 320mm. If its length increases by 10mm and its breadth decreases by 10mm then it its area will be 32 less. Calculate the length and breadth of the original rectangle. (20)

Answer 1

The length of the original rectangle is 73.4 mm.

The breadth of the original rectangle is 86.6 mm.

Explanation:

Given : The perimeter of a rectangle is 320 mm. If its length increases by 10 mm and its breadth decreases by 10 mm then it its area will be 32 less.

To find : Calculate the length and breadth of the original rectangle ?

Solution :

The area of the rectangle is
`A=L* B`

Let the length of the rectangle be 'x'

The breadth of the rectangle be 'y'

The area is
`A=xy`

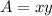
Now, length increases by 10 mm i.e. L=x+10

breadth decreases by 10 mm i.e. B=y-10

The new area is
`A_n=(x+10)(y-10)`

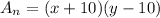
According to question,

`A-A_n=32`

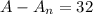
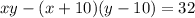`xy-(x+10)(y-10)=32` ......(1)

The perimeter of a rectangle is 320 mm.

i.e.
`P=2(L+B)`

`320=2(x+y)`

`x+y=160`

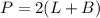
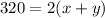
`x=160-y` .....(2)

Substitute the value of y from eqn (2) in (1),

`y(160-y)-(160-y+10)(y-10)=32`

`160y-y^2-(170-y)(y-10)=32`

`160y-y^2-(180y-1700-y^2)=32`

`160y-y^2-180y+1700+y^2=32`

`1700-20y=32`

`20y=1732`

`y=(1732)/(20)`

`y=86.6`

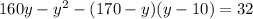
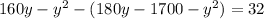
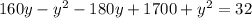
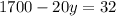
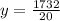
Substitute in (2),

`x=160-86.6`

`x=73.4`

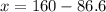
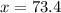
The length of the original rectangle is 73.4 mm.

The breadth of the original rectangle is 86.6 mm.