Question

The speed of sound in air is 345 m/s. A tuning fork vibrates above the open end of a sound resonance tube. If sound waves have wavelengths 63-cm in the tube, what is the frequency (in Hertz) of the tuning fork? Never include units with a numerical answer.

Answer 1

To develop this problem it is necessary to apply the concept of Frequency based on speed and wavelength.

According to the definition the frequency can be expressed as

`f = (v)/(\lambda)`

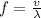
Where,

v = Velocity

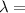`\lambda =` Wavelength

Our value are given by,

v = 345m/s

`\lambda = 63cm`

Replacing

`f = (345)/(0.63)`

`f = 547.61Hz`

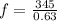
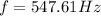
Therefore the frequency of the tuning fork is 547.61Hz